3D-printed Conformations of Knots through 7 Crossings

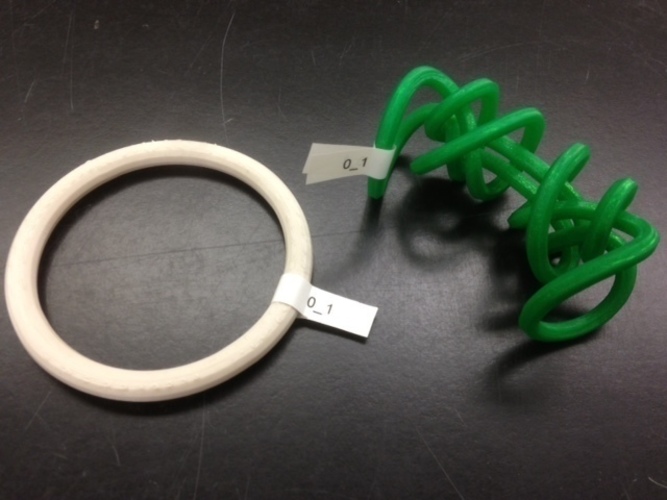
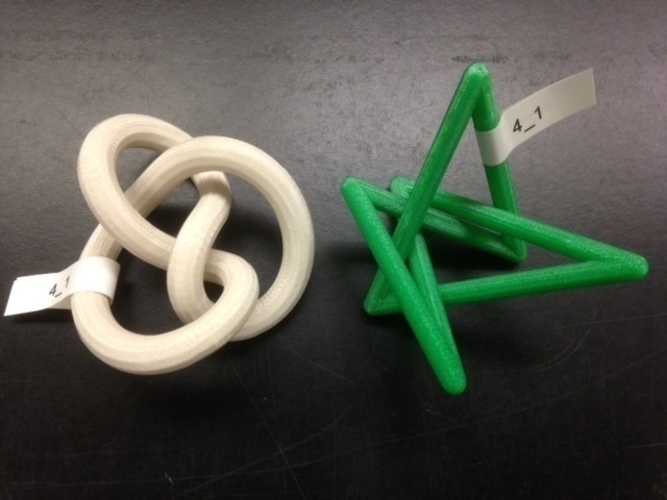
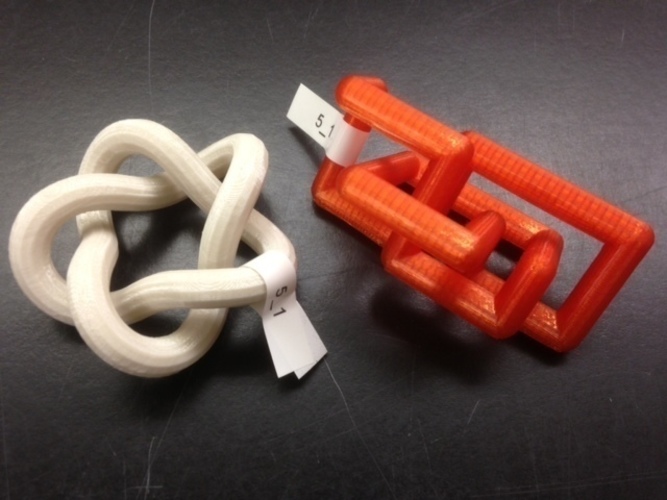
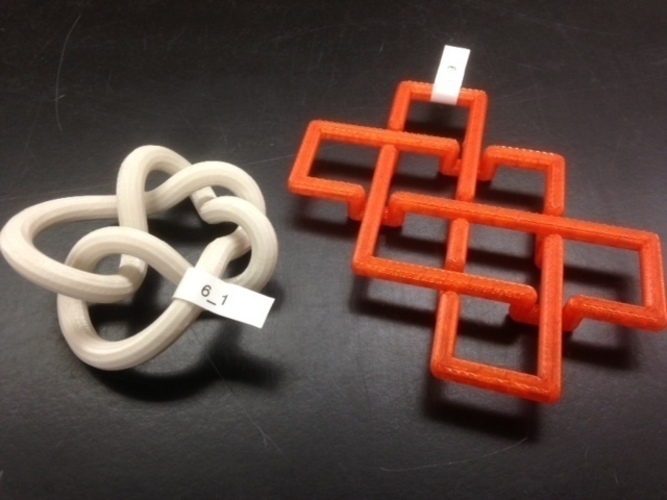
Knots are embeddings of circles in 3-dimensional space, but they are typically studied in terms of their projections into 2-dimensional space. We can use 3D printing to study knots in a more 3-dimensional way.

In this series we present 3D printed conformations of the fifteen knots through seven crossings, including stick, Lissajous, lattice, torus, petal, and pretzel conformations. Constructing a 3D knot model that is actually printable can challenging; to 3D print the knot conformations through seven crossings, we used a combination of Mathematica, Blender, Tinkercad, Knotplot, SeifertView, and OpenSCAD.

For detailed information about the mathematics and the design behind these models, see the MakerHome blog: 

0_1 at Day 267

3_1 at Day 268 

4_1 at Day 269 

5_1 at Day 272 

5_2 at Day 273 

6_1 at Day 275 

6_2 at Day 276 

6_3 at Day 285 

7_1 at Day 286 

7_2 at Day 289 

7_3 at Day 290 

7_4 at Day 291 

7_5 at Day 295 

7_6 at Day 296 

7_7 at Day 299

=====

Twitter:  twitter.com/mathgrrl

Hacktastic blog:  www.mathgrrl.com/hacktastic

Shapeways geekhaus store:  www.shapeways.com/shops/mathgr... 

This design and all associated pictures and files are licensed under the Creative Commons Attribution Non-Commercial Share Alike license. If you want to use designs, images, or files outside of the terms of this license, please email [email protected].