Calculus: Introduction to Solids of Revolution

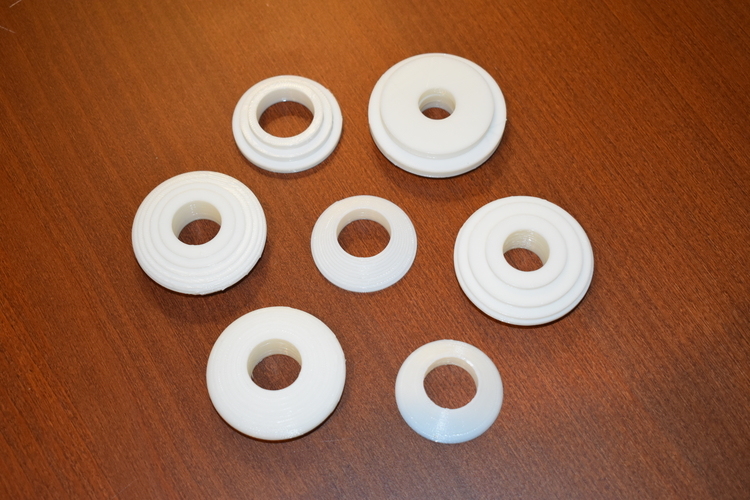
This lesson and 3D models are intended for students in calculus, and to demonstrate the methods of using shells and washers to find the volume of Solids of Revolution. The models can be used with a pre-existing lesson plan. I printed the models with my TAZ 5. I used supports (and a dremel) for those with large overhangs, and no supports for the others. They printed pretty well. 

Lesson Objectives:

Provide a meaningful model and explanation to students about how area and volume can be approximated by the sums of parts (thin cross sections of a solid, or thin slices of an area), and with a thickness (dx) approaching zero; ultimately, how integration can be used to find the volume of a solid.

Allow calculus students to visualize the methods of using washers or shells to calculate the volume of areas swept out about an axis – called Solids of Revolution. 

This lesson plan doesn't include a way to assess the students' understanding of the topic or use of the models, as the lesson plan and models are only intended to be used as an introduction to the subject, and to aid visualization of each method. 

Included Models:

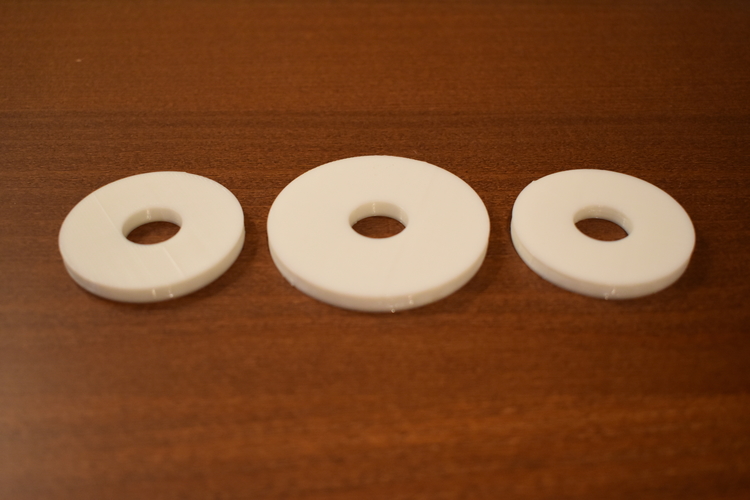
Washers/Disks: 

- A three piece separable/stackable model to demonstrate the method. 

- Solids of revolution to be found via method of washers, with decreasing thicknesses (dx), approximating volume with increasing accuracy. 

Shells: 

- A three piece model that fits together to demonstrate the method, along with a beam that demonstrates how the volume of each shell is approximated by the circumference of each shell and a thickness (dx). 

- Solids of revolution to be found via method of shells, with decreasing shell thickness (dx, or dr), approximating volume with increasing accuracy.